Infrared Camera Accuracy and Uncertainty in Plain Language

It’s tough to trust measurements from instruments when you don’t have a clear understanding of how their sensitivity and accuracy is derived, and many times infrared cameras fall in this category. Additionally, discussions of infrared camera measurement accuracy typically involve complex terms and jargon that can be confusing and misleading. This can ultimately prompt some researchers to avoid these tools altogether. However, by doing so, they miss out on the potential advantages of thermal measurement for R&D applications. In the following discussion, we strip away the technical terms and explain measurement uncertainty in plain language, providing you with a foundation that will help you understand IR camera calibration and accuracy.

Camera Accuracy Specs and the Uncertainty Equation

You’ll notice that most IR camera data sheets show an accuracy specification such as ±2ºC or 2% of the reading. This specification is the result of a widely used uncertainty analysis technique called “Root‐Sum‐of‐Squares”, or RSS. The idea is to calculate the partial errors for each variable of the temperature measurement equation, square each error term, add them all together, and take the square root. While this equation sounds complex, it’s fairly straightforward. Determining the partial errors, on the other hand, can be tricky.

“Partial errors” can result from one of several variables in the typical IR camera temperature measurement equation, including:

• Emissivity
• Reflected ambient temperature
• Transmittance
• Atmosphere temperature
• Camera response
• Calibrator (blackbody) temperature accuracy

Once reasonable values are determined for the “partial errors” for each of the above terms, the overall error equation will look like this:

Where the ΔT1, ΔT2, ΔT3, etc are the partial errors of the variables in the measurement equation.

Why do this? It turns out that random errors sometimes add in the same direction, taking you farther from the true value, while other times they add in opposite direction and cancel each other out. Taking the RSS gives you a value that is most appropriate for an overall error specification. This has historically been the specification shown on FLIR camera data sheets.

It’s worth mentioning that the calculations discussed so far are only valid if the camera is bein used in the lab or at short range (less than 20 meters) outside. Longer ranges will introduce uncertainty in the measurement because of the atmospheric absorption and to a lesser extent, its emission. When a camera R&D engineer performs an RSS analysis for almost any modern IR camera system under lab conditions, the resulting number is around ±2ºC or 2% – making this a reasonable accuracy rating to use in camera specifications.

However, practice shows us that high performance cameras, such as the FLIR X6900sc, give much better results than economical cameras like the FLIR E40, so we still have some work to do to better explain this observation.

Laboratory Measurements and ±1°C or 1% Accuracy

In this section, we take a look at the temperature measurements a camera actually produces when looking at an object of known emissivity and temperature. Such an object is commonly referred to as a “blackbody”. You may have heard this term before in reference to the theoretical concept of an object with known emissivity an temperature. It is also used to describe a piece of lab equipment which closely emulates this concept. FLIR’s calibration lab with quarter circle of at least 2 cavity blackbodies is shown below.

Laboratory measurements of uncertainty involve pointing a calibrated camera at a calibrated blackbody and plotting the temperature over a period of time. Despite the careful calibrations, there will always be some random error in the measurement. The resulting data set can be quantified for accuracy and precision. Figure 2, below, demonstrates the results from calibrated blackbody measurement.

The below plot shows more than two hours of data from a FLIR A325sc camera looking at a 37ºC blackbody at a range of 0.3 meter in an indoor environment. The camera recorded the temperature once per second. The data plotted is the average of all pixels in the image. A histogram of this data would make it clearer, but most of the data points were between 36.8ºC and 37ºC. The widest ranging temperatures recorded were 36.6ºC and 37.2ºC.

Looking at this data, it would be tempting to claim an expected accuracy of 0.5ºC for the average of all the pixels. One could even claim ±1ºC for the FLIR A325sc and any other camera using the same detector. However, one could also argue that the above graph shows an average of all of the pixels and may not be representative of an individual pixel.

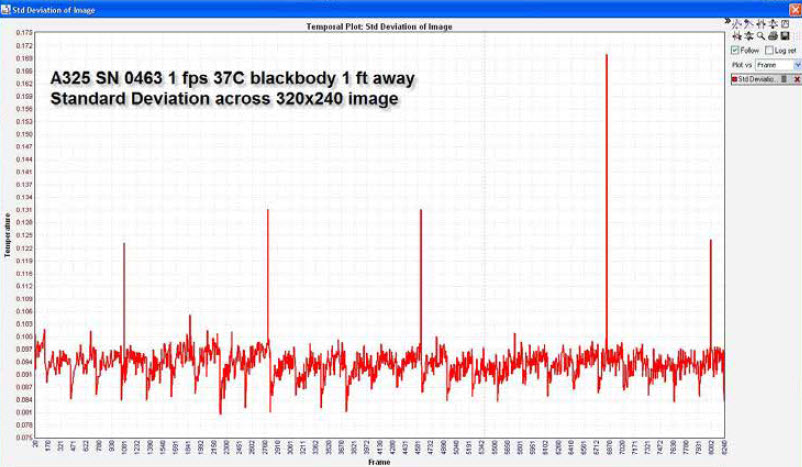
One way of knowing how well all of the pixels agree with each other is to look at standard deviation versus time. This is represented in Figure 3. The graph shows that the typical standard deviation is less than 0.1ºC. The occasional spikes to around 0.2ºC are a result of the camera’s 1‐point update, a type of self‐calibration procedure that all microbolometer‐based cameras must perform periodically.

So far we have discussed collecting data from uncooled microbolometer cameras. How will the results differ for a high-performance quantum detector camera?

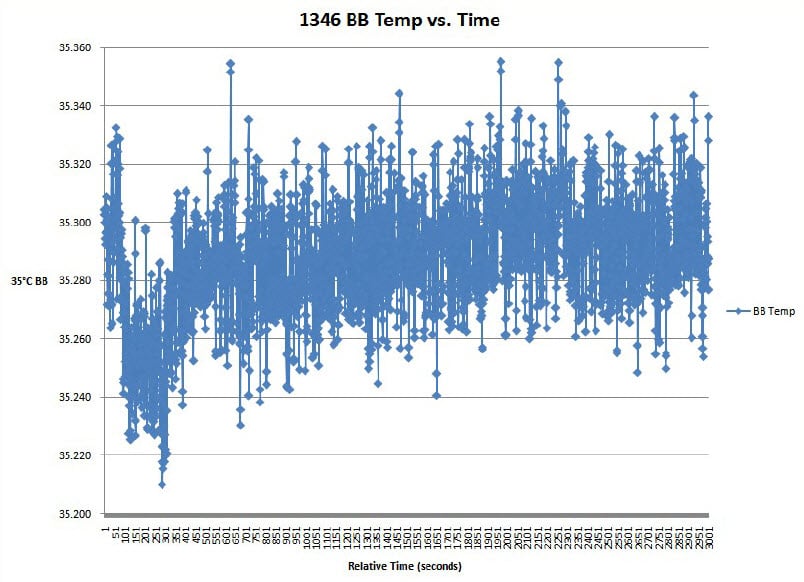
Figure 4 shows the response of a typical 3‐5 μm camera with an Indium Antimonide (InSb) detector, such as the FLIR X6900sc. That camera’s documentation shows the accuracy tested at ±2ºC or 2%. On the graph below, you can see the results fall well within those specifications: the accuracy reading on that day was around 0.3ºC and the precision reading was around 0.1ºC. But why is the offset error at 0.3ºC? This could be cause by the calibration of the blackbody, the calibration of the camera, or any of the partial error terms mentioned in section 2. Another possibility is the camera was simply warming up at the beginning of the measurement. If the optics or the inside of the camera body are changing temperature, they may offset the temperature measurement.

The conclusion we can draw from these two calibration tests is that both microbolometer and photon‐counting quantum detector cameras can be factory calibrated to provide accuracies of less than 1ºC when looking at 37ºC objects of known emissivity under typical indoor environmental conditions.

Ambient Temperature Compensation

One of the most critical steps in factory calibrations is ambient temperature compensation. Infrared cameras – whether thermal or quantum detecting – respond to the total infrared energy falling on the detector. If the camera is designed well, most of this energy will be from the scene: very little results from the camera itself. However, it’s impossible to completely Figure 2 – Typical FLIR A325sc camera response when looking at a 37ºC blackbody Figure 3 – Standard Deviation of typical A325sc when looking at 37ºC blackbody Figure 4– Response of a typical InSb camera looking at a 35ºC blackbody eliminate the contribution from the materials surrounding the detector and the optical path. Without proper compensation, any changes to the temperature of the camera body or lenses will significantly alter the temperature readings the camera provides.

The best method for achieving ambient temperature compensation is to measure the temperature of the camera and optical path in up to three different locations. The measurement data is then included in the calibration equation. This can ensure accurate readings through the entire range of operating temperatures (typically ‐15ºC to 50ºC). This is particularly important for cameras that will be used outdoors or otherwise subjected to temperature swings.

Even with Ambient Temperature Compensation, it’s important to allow the camera to fully warm up before making critical measurements. Also, keep the camera and optics out of direct sunlight or other sources of heat. Changing the temperature of the camera and optics will have an adverse effect on measurement uncertainty.

We should note that not all camera makers include ambient temperature compensation in their calibration process. By not properly compensating for ambient temperature drift, the data from these cameras could show significant inaccuracies – as much as 10oC or more. Therefore, be sure to ask about calibrations and how they’re performed before investing in an IR camera.

Other Measurement Considerations

While not directly related to camera calibration, considerations such as emissivity and spot size can impact camera accuracy. An incorrect emissivity setting or improper testing conditions will affect the camera’s ability to measure your subject correctly.

Emissivity – or an object’s ability to emit rather than reflect infrared energy – must be properly accounted for. This means taking the time to determine the emissivity of your subject and entering that information in the camera. It also means paying attention to whether the subject is completely reflective and taking steps to resolve that (e.g. coating the surface with nonreflective paint) before measuring. All FLIR cameras provide a means of defining an appropriate emissivity. If you make a mistake, all FLIR R&D software allows you to change the emissivity in during analysis (live viewing or post‐analysis). This can typically be done on a whole image or a region by region basis.

Another factor to consider is the spot size, or how much area each pixel covers on your target. Let’s say an A325sc with a default 25 degree lens is measuring a lit match that is 60 feet away. Each pixel covers about an inch square area of the total scene. But a match head is only about 1/8” square – much smaller than the pixel covering it. Nearly all of the infrared energy striking that pixel actually comes from the area behind the match ember. Only 1/64ths of the contribution is coming from the ember we intended to measure. If the background is at room temperature, the camera will severely under‐report the temperature of the ember.

The solution would be to attach a telescopic optic to the camera, or simply move it closer to the target. Either would bring the pixel size closer to a 1:1 ratio with the ember. If we want the closest to absolute temperature accuracy, we must ensure that the smallest object of interest is fully subtended by at least a 10 x 10 pixel grid. However, even considering the spot size to be a single pixel or a 3 x 3 pixel grid will get you very close to true measurement.

Conclusion

As we have seen, the RSS uncertainty analysis technique allows us to determine the accuracy of infrared cameras, and that these cameras may have, at most, a 2ºC margin of error. With proper calibration and attention to factors such as ambient temperature, emissivity, and spot size, the possible margin of error can be less than 1ºC.

One final note: the information presented in this paper was primarily written with factory calibrated infrared cameras in mind. While the physics are applicable to user calibrations, the tools and methods needed for user calibrations vary depending on the system being discussed. In addition, being able to perform a good user calibration would allow you to perform a custom uncertainty analysis – making generalized specifications discussed in this paper less relevant.